The Fractalist
By
Adam Kirsch
Tablet Magazine, October 25, 2012
Edited by Andy Ross
Benoit Mandelbrot gained fame for his book
The Fractal Geometry of Nature (1982).
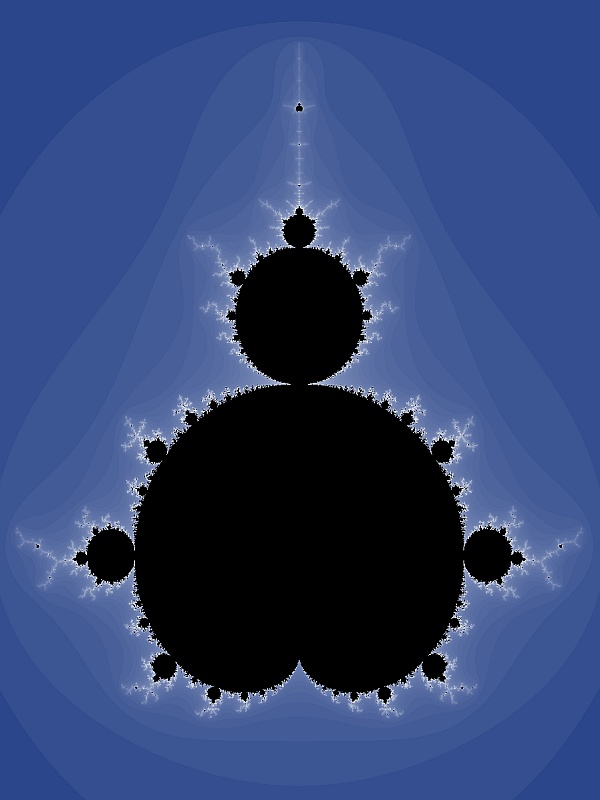
The Mandelbrot set is
generated as follows: Pick a constant c and let the original z be at the
origin of the plane; replace z by z times z; add the constant c; repeat. A
graph of the set produces an image of strange and fantastic complexity. It
is a fractal: it is self-similar; it repeats its own pattern on every scale.
This kind of pattern is common in nature. Mandelbrot coined the term
fractal after consulting his son's Latin dictionary and finding the word
fractus (broken). As he claimed in 1982: "Clouds are not spheres, mountains
are not cones, coastlines are not circles, and bark is not smooth, nor does
lightning travel in a straight line." Mandelbrot developed mathematical
tools to measure such complexity. He says his gift was an intuitive ability
to "see" complex shapes.
The Fractalist is Mandelbrot's memoir. Its first half is compelling. It
describes his childhood and adolescence as a Jew in wartime Europe. He was
born in Warsaw in 1924 and raised in a modernizing Jewish family where
Polish was the language of choice. Poland was a difficult place for Jews.
His father emigrated to Paris in 1931, and five years later his family
followed. The move came just in time. A few years later, they would have
been trapped in the Holocaust.
Life in France was not easy. The
family was extremely poor and lived in a Paris slum. But Mandelbrot's
parents believed in the French school system. After the fall of France in
1940, the Mandelbrots fled south. Academic brilliance won him special
consideration: "Xenophobia lost, meritocracy won." Mandelbrot won a place at
the École Polytechnique. His entry exam score was the highest in France.
From then on he was made.
The second half of the memoir relates
Mandelbrot's distinguished academic and professional career. He spent most
of his adult life in the United States as a researcher at IBM. This half of
the story makes Mandelbrot seem vain. This is a man who decided early in
life to revolutionize our picture of the world: "In my Keplerian quest I
faced many challenges. The good news is that I succeeded."
Benoit Mandelbrot
"I have never done anything like others", Mandelbrot once said. And indeed
these memoirs show it. He really managed to do everything on his own terms.
It was not easy for him.
Consider his huge insight about the world
around us. Triangles, squares, and circles seem to exist in our textbooks
more than reality. Thus was born fractal geometry, a general theory of
"roughness". Mandelbrot uncovered simple rules used by nature (and men)
that, thanks to repetition, by smaller parts that resemble the whole,
generate these seemingly complex and chaotic patterns.
Self-taught
and fiercely independent, he thought in images and passed the entrance exam
of the top school of mathematics without solving equations. He produced the
famous Mandelbrot set when he was in his fifties and got tenure at Yale when
he was 75. The top prize in mathematics was recently given for solving one
of his sub-conjectures.
Mandelbrot, while a bit of a loner, had
perhaps more cumulative influence than any other single scientist in
history, with the only close second Isaac Newton. His contributions affected
physics, engineering, arts, medicine, biology, etc. But he was unheeded in
economics, where he proved in the 1960s that financial theories vastly
underestimate market risk and need total revamping.
I met him when he
was in his late seventies, as he was writing these memoirs. He was the only
teacher I ever had, the only person for whom I have had intellectual
respect.
By Jim Holt
The New York Review of Books, May 23, 2013
Edited by Andy Ross
Consider the cauliflower. Observe the way its head is composed of florets.
Each one of those florets looks like a little head of cauliflower, with its
own subflorets. Now pull off a subfloret and observe the way … continue this
process. Each part is similar to the whole.
Benoit Mandelbrot created
a new branch of geometry based on such self-similarity. The mathematics of
self-similarity also covers kindred matters like turbulence, noise,
clustering, and chaos. Mandelbrot worked as a researcher for IBM in upstate
New York. There he coined the word "fractal" to designate self-similar
forms. In 1980 he discovered the Mandelbrot set. He also created fractal
financial models showing that stock and currency markets were far riskier
than investors supposed.
Agin and again, Mandelbrot found simplicity
and even beauty where others saw irredeemable messiness. He relied on visual
insight: "When I seek, I look, look, look."
Mandelbrot was born in
1924 into a Jewish family that lived in the Warsaw ghetto. His father sold
ladies' hosiery and his mother was a dentist. His uncle Szolem was a
mathematician of international rank. After his father's business collapsed,
the family left Poland for Paris, traveling across Nazi Germany in a
padlocked train.
In Paris, Mandelbrot found he could see simple
shapes behind complicated equations and conceived his "Keplerian quest".
Three centuries earlier, Johannes Kepler had seen the simple geometry of an
ellipse behind complicated equations for the motions of the planets.
Mandelbrot "came to worship" Kepler's achievement and aspired to do
something similar.
Uncle Szolem urged Mandelbrot to attend the École
Normale Supérieure. At the time, its mathematics was dominated by the
Bourbaki cult, led by André Weil, whose aim was to purify mathematics, to
rebuild it on perfectly logical foundations untainted by physical or
geometrical intuition. Mandelbrot found the cult "positively repellent". The
cultists cut off mathematics from natural science to make a sort of logical
theology. They regarded geometry as a dead branch of mathematics. Mandelbrot
resigned. A few adventures later, he became a grad student at the University
of Paris.
One day, Uncle Szolem gave him a paper about Zipf's law.
This law concerns the frequency of different words in written texts. Zipf
ranked the words and plotted their frequency. The curve plunged sharply at
first and then leveled off into a long and gently sloping tail. A few
hundred words do almost all the work, and the great majority are hardly used
at all. The law was a simple yet precise relation between rank and
frequency.
The same basic principle turns out to be valid for a great
variety of phenomena. All are power law distributions. Power laws apply, in
nature or society, where there is extreme inequality or unevenness: where a
high peak is followed by a long tail. In such cases, the notion of an
average is meaningless.
Mandelbrot recalled his encounter with the
law as a eureka moment: "I saw that it might be deeply linked to information
theory and hence to statistical thermodynamics — and became hooked on power
law distributions for life." He wrote his Ph.D. thesis on Zipf's law. Much
later, he became known as "the father of long tails".
In the United
States, Mandelbrot discovered Norbert Wiener and John von Neumann.
Mandelbrot saw them both as "made of stardust" and worked with them.
Returning to Europe, Mandelbrot got married and stayed a while in Paris,
then accepted a job at IBM in Yorktown Heights, New York. There he found his
scientific home. The director of research told him: "We can easily afford a
few great scientists doing their own thing."
Visiting Harvard to
lecture on power laws and the distribution of wealth, Mandelbrot was struck
by a diagram he saw on a chalkboard. The curve looked like the one he was
about to present in his lecture, yet it described price jumps on the New
York Cotton Exchange. The curb was inconsistent with the orthodox efficient
market hypothesis. Mandelbrot later studied the data and found a power law
at work: "The very heart of finance is fractal."
The fractal model of
financial markets has yet to catch on. Mandelbrot was pushed out of
economics and returned to IBM. In 1987, he moved to Yale and finally got
tenure in 1999, at the age of 75. Most finance professors still cling to the
efficient market hypothesis. If Mandelbrot is right, reliance on orthodox
models is dangerous. And so it proved in 2008.
Mandelbrot made the
most momentous discovery of his career at Harvard in 1980. He taught a
course on how fractal ideas could help in classical mathematics. This led
him to take up complex dynamics, which had been studied in Paris decades
earlier but led to geometrical forms that were far too complicated for that
time.
Mandelbrot had access to a computer. He used a new Vax
supermini to zoom in on a geometrical figure generated by a very simple
formula. The set now named in his honor is a thing of infinite beauty. Its
detailed geometry, still far from fully understood, encodes an infinite
bestiary of chaotic processes. An endlessly complex object arises from a
simple formula.
For Mandelbrot, the world we live in is an infinite
sea of complexity. Yet it contains two islands of simplicity. The Euclidean
simplicity of smooth forms was discovered by the ancients. The fractal
simplicity of self-similar roughness was largely discovered by Mandelbrot
himself. He detected a new Platonic essence shared by a wide assortment of
particulars, from the cauliflower to the Mandelbrot set.
AR The Mandelbrot set and the 1982 book amazed me in London in the Eighties. The mathematics of it all still amazes me.
|
|
|