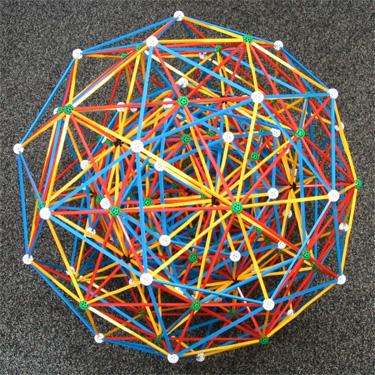
Vertex-edge graph of the E8 polytope
An Exceptionally Simple Theory of Everything
By Roger Highfield
Daily Telegraph, November 14, 2007
Edited by Andy Ross
Garrett Lisi, 39, completed his doctorate in theoretical physics in 1999 at
the University of California, San Diego, but has no university affiliation
and spends most of the year surfing in Hawaii. In winter, he heads to
Nevada, where he snowboards.
His proposal is remarkable because it
does not require highly complex mathematics, it does not require more than
one dimension of time and three of space, and it may even be testable, since
it predicts a host of new particles.
Lee Smolin at the Perimeter
Institute for Theoretical Physics in Waterloo, Ontario, Canada, describes
Lisi's work as "fabulous". "It is one of the most compelling unification
models I've seen in many, many years," he says.
"Some incredibly
beautiful stuff falls out of Lisi's theory," adds David Ritz Finkelstein at
the Georgia Institute of Technology, Atlanta. "This must be more than
coincidence and he really is touching on something profound."
He has
high hopes that his new theory could provide a radical new explanation for
the Standard Model, which weaves together three of the four fundamental
forces of nature: the electromagnetic force, the strong force, and the weak
force. His model also takes account of gravity.
Lisi's inspiration
lies in the most elegant and intricate shape known to mathematics, called
E8, only fully understood by mathematicians earlier this year. E8 is at the
heart of many bits of physics. One interpretation of why we have such a
quirky list of fundamental particles is because they all result from
different facets of the symmetries of E8.
Lisi realised that he could
find a way to place the various elementary particles and forces on the 248
points of E8. What remained was 20 gaps which he filled with notional
particles, for example those that some physicists predict to be associated
with gravity.
Physicists have long puzzled over why elementary
particles appear to belong to families, but this arises naturally from the
geometry of E8, he says. So far, all the interactions predicted by the
complex geometrical relationships inside E8 match with observations in the
real world. "How cool is that?" he says.
The crucial test of Lisi's
work will come only when he has made testable predictions. Lisi is now
calculating the masses that the 20 new particles should have, in the hope
that they may be spotted when the Large Hadron Collider starts up.
Is this the fabric of the universe?
By Roger Highfield
Daily Telegraph, March 19, 2007
Edited by Andy Ross
Mathematicians today unveiled the result of an arcane investigation of a
group called E8. The combined assault on what is described as "one of the
largest and most complicated structures in mathematics" required the effort
of 18 mathematicians from America and Europe for an intensive four-year
collaboration.
"The group of symmetries of this strange geometry
called E8 is one of the most intriguing structures that Nature has left for
the mathematician to play with," commented Professor Marcus du Sautoy of
Oxford University. "Most of the time mathematical objects fit into nice
patterns that we can order and classify. But this one just sits there like a
huge Everest."
For the feat, the team used a mix of theoretical
mathematics and intricate computer programming to map E8, which is an
example of a Lie group. Lie groups were invented by the 19th century
Norwegian mathematician Sophus Lie to study symmetry. Underlying any
symmetrical object is a Lie group.
"E8 was discovered over a century
ago, in 1887, and until now, no one thought the structure could ever be
understood," said Professor Jeffrey Adams, Project Leader, at the University
of Maryland. "This groundbreaking achievement is significant both as an
advance in basic knowledge, as well as a major advance in the use of large
scale computing to solve complicated mathematical problems."
"This is
an exciting breakthrough," said Professor Peter Sarnak at Princeton
University. "Understanding and classifying the representations of E8 and Lie
groups has been critical to understanding phenomena in many different areas
of mathematics and science including algebra, geometry, number theory,
physics and chemistry."
The goal was to describe all the possible
representations of E8. These representations are extremely complicated, but
mathematicians describe them in terms of basic building blocks. The new
result is a complete list of these building blocks for the representations
of E8, and a precise description of the relations between them, all encoded
in a matrix, or grid, with 453,060 rows and columns. In all, there are
205,263,363,600 entries, each one a polynomial.
The result of the E8
calculation, which contains all the information about E8 and its
representations, is 60 gigabytes in size. The computation required
sophisticated new mathematical techniques and computing power not available
even a few years ago.
"This is an impressive achievement," said
Hermann Nicolai, Director of the Albert Einstein Institute in Potsdam,
Germany.
E8
Edited by Andy Ross
In mathematics, E8 is the name given to a family of closely related
structures. In particular, it is the name of some exceptional simple Lie
algebras as well as that of the associated simple Lie groups. It is also the
name given to the corresponding root system, root lattice, and various
related groups. E8 was formulated between the years of 1888 and 1890 by
Wilhelm Killing.
The designation E8 comes from Wilhelm Killing and
Élie Cartan's classification of the complex simple Lie algebras, which fall
into four infinite families and five exceptional cases. The E8 algebra is
the largest and most complicated of these exceptional cases.
E8 has
rank 8 (the maximum number of mutually commutative degrees of freedom) and
dimension 248 (as a manifold). The vectors of the root system are in eight
dimensions. The Weyl group of E8, which acts as a symmetry group of the
maximal torus by means of the conjugation operation from the whole group, is
of order 696,729,600.
E8 is unique among simple Lie groups in that
its non-trivial representation of smallest dimension is the adjoint
representation (of dimension 248) acting on the Lie algebra E8 itself.
The complex Lie group E8 of complex dimension 248 can be considered as a
simple real Lie group of (real) dimension 496, which is simply connected,
has maximal compact subgroup the compact form of E8, and has an outer
automorphism group of order 2 generated by complex conjugation. As well as
the complex Lie group of type E8, there are three real forms of the group,
all of real dimension 248.
The coefficients of the character formulas
for infinite dimensional irreducible representations of E8 depend on some
large square matrices consisting of polynomials. These matrices were
computed after four years of collaboration by a group of 18 mathematicians
and computer scientists, led by Jeffrey Adams, with much of the programming
done by Fokko du Cloux. The result was announced in March 2007.
The
compact real form of E8 is the isometry group of a 128-dimensional
Riemannian manifold known informally as the octo-octonionic projective plane
because it can be built using an algebra that is the tensor product of the
octonions with themselves.
A root system of rank r is a particular
finite configuration of vectors, called roots, which span an r-dimensional
Euclidean space and satisfy certain geometrical properties. In particular,
the root system must be invariant under reflection through the hyperplane
perpendicular to any root.
The E8 root system is a rank 8 root system
containing 240 root vectors spanning R8. It is irreducible in the sense that
it cannot be built from root systems of smaller rank. Each of the root
vectors in E8 have equal length.
The E8 Lie group has applications in
string theory and supergravity. The group E8 x E8 (the Cartesian product of
two copies of E8) serves as the gauge group of one of the two types of
heterotic string and is one of two anomaly-free gauge groups that can be
coupled to N = 1 supergravity in 10 dimensions. E8 is the U-duality group of
supergravity on an eight-torus (in its split form).
One way to
incorporate the standard model of particle physics into heterotic string
theory is the symmetry breaking of E8 to its maximal subalgebra SU(3) x E6.
In 1982, Michael Freedman used the E8 lattice to construct an example of
a topological 4-manifold, the E8 manifold, which has no smooth structure.
An Exceptionally Simple Theory of Everything
By Antony Garrett Lisi
November 6, 2007
This theory claims to unify all fields of the standard model with gravity
using a 248-point lattice of E8 geometry. The title is a mathematical pun on
E8's classification as both a simple group and an exceptional group.
In its current form, the theory does not reproduce the particle content of
the Standard Model (SM) and it is not a quantum theory. Lisi claims that it
will be testable soon using the Large Hadron Collider which is slated to
come on line in May 2008. However, his paper contains no calculations for
particle masses, and it is not clear that such calculations could be done
even in principle.
Sabine Hossenfelder, of the Perimeter Institute for
Theoretical Physics, said the model:
— Does not naturally lead to a
unification of the SM interactions with gravity (he has to choose the action
by hand that contains both).
— Does not allow us to understand quantum
gravity (since nothing is said about quantization).
— Does not
explain the parameters in the SM (it does not yet have a mechanism for
symmetry breaking).
— Does not explain the cosmological constant or its
value.
— Does not explain the hierarchy problem.
— Does not explain
why we live in a spacetime with 4 dimensions.
Overall she thinks the
paper has potential.
New Scientist 2-min video introduction to the theory
Entertaining physicist blog rant against the theory
AR This theory animated me greatly in 2008.